what is the value of 7 to the fifth power

Exponents Calculator or e calculator is used in solving exponential forms of expressions. It is also known as raised to the power calculator.

Backdrop of exponents calculator:

This calculator solves bases with both negative exponents and positive exponents. It also provides a footstep by step method with an accurate answer.

What is an exponent?

 An exponent is a pocket-size number located in the upper, right-hand position of an exponential expression (base exponent), which indicates the ability to which the base of the expression is raised.

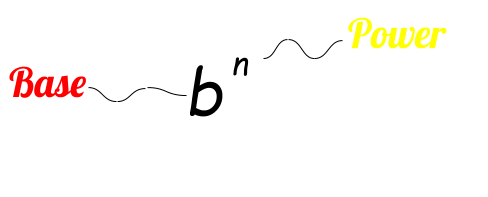
The exponent of a number shows you lot how many times the number is to exist used in a multiplication. Exponents do non have to exist numbers or constants; they can be variables.

They are ofttimes positive whole numbers, but they tin can be negative numbers, fractional numbers, irrational numbers, or complex numbers. Information technology is written every bit a small number to the right and above the base number.

Types:

There are basically 2 types of exponents.

• Positive exponent

A positive exponent tells how many times a number is needed to be multiplied by itself. Apply our exponent computer to solve your questions.

• Negative exponent

A negative exponent represents which fraction of the base of operations, the solution is. To simplify exponents with power in the class of fractions, utilize our exponent reckoner.

Example:

Calculate the exponent for the 3 raised to the ability of 4 (3 to the power of 4).

It means = iii⁴

Solution:

3*3*iii*3 = 81

4 to the 3rd ability = 81

Therefore the exponent is 81

2 raised to the power calculator.

Example:

What is the value of exponent for ii heighten to ability nine (two to the 9th power)

It means = 2⁹

Solution:

2*2*two*2*2*2*two*2*2 = 512

ii to the ninth power = 512

Therefore the exponent is 512.

Instance :

How do y'all calculate the exponents of 5,6,seven to the ability of 4?

It means = 5^iv, 6^iv, vii⁴

Solution:

v*5*5*5 = 625

6*6*6*6 = 1296

7*7*seven*7 = 2401

Therefore the exponents are 625, 1296, 2401.

How to calculate the nth power of a number?

The nth power of a base, let'southward say "y", ways y multiplied to itself nth time. If nosotros are to detect the fifth power of y, it is y*y*y*y*y.

Some other solutions for the nth power calculator are in the following tabular array.

0.ane to the power of three	0.00100
0.five to the ability of 3	0.12500
0.5 to the power of 4	0.06250
1.ii to the ability of 4	two.07360
1.02 to the 10th power	1.21899
1.03 to the 10th power	1.34392
1.2 to the power of 5	ii.48832
one.4 to the 10th power	28.92547
1.05 to the power of 5	i.27628
1.05 to the 10th ability	ane.62889
1.06 to the 10th power	1.79085
2 to the tertiary power	8
ii to the power of 3	8
two raised to the power of 4	16
2 to the ability of 6	64
2 to the power of seven	128
2 to the 9th power	512
2 to the tenth power	1024
2 to the 15th power	32768
two to the 10th power	1024
ii to the power of 28	268435456
3 to the power of 2	ix
3 to the three power	27
3 to the 4 power	81
3 to the eighth power	6561
3 to the 9th power	19683
3 to the 12th ability	531441
3 to what ability equals 81	iii4
4 to the power of iii	64
4 to the power of 4	256
iv to the ability of vii	16384
7 to the power of iii	343
12 to the 2d power	144
two.5 to the power of 3	fifteen.625
12 to the power of 3	1728
10 exponent 3	1000
24 to the second power (242)	576
10 to the power of three	1000
3 to the ability of 5	243
6 to the power of 3	216
nine to the power of 3	729
9 to the power of ii	81
x to the power of 5	100000

Exponent Rules:

Learning the exponent rules forth with log rules tin can make maths actually easy for understanding. In that location are 7 exponent rules.

• Zero Belongings of exponent:

 It means if the power of a base of operations is cypher then the value of the solution volition be ane.

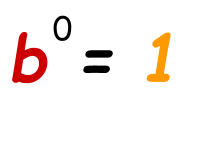
Case: Simplify 5⁰.

In this question, the ability of base is null, then according to the zero belongings of exponents, the answer of this non zero base is 1. Hence,

5⁰= 1

• Negative Property of exponent:

It ways when the power of base is a negative number, then afterwards multiplying nosotros will take to find the reciprocal of the answer.

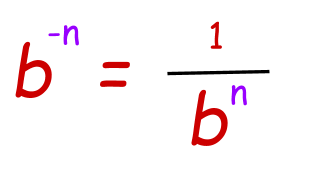
Case: Simplify i/3^-2.

Nosotros volition first make the power positive by taking reciprocal.

i/3^-2=3²

3ⁱⁱ = 9

• Production Property of exponent:

When two exponential expressions having the aforementioned not naught base of operations and different powers are multiplied, then their powers are added over the same base.

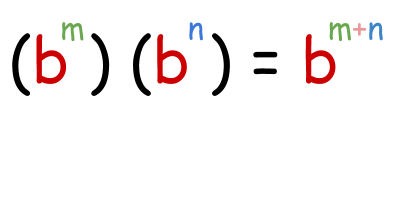
Example: Solve (2⁶)(2ⁱⁱ).

Every bit it is obvious, bases are the same then powers are to exist added. Now

(two^{half dozen})(two²) = 2^6+two

2⁸ =two*2*2*two*2*2*2*ii

=256

• Caliber Property of exponent:

It is the opposite of the product property of exponent. When two aforementioned bases having unlike exponents are required to be divided, so their powers are subtracted.

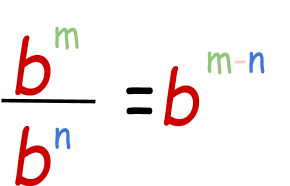
Case: Simplify 3⁷ /three²

3⁷/ 3ⁱⁱ=3^7-2

35=3*3*3*3*iii

= 243

• Power of a Ability Property:

When an exponent expression further has power, and then firstly you need to multiply the powers and then solve the expression.

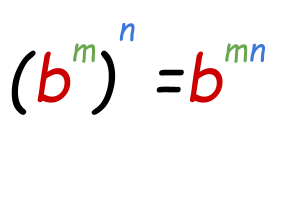
Example: Solve: ( x²)ⁱⁱⁱ.

Keeping in view the power of ability property of exponents, we will multiply powers.

(10ⁱⁱ)³=x^two*three

= x^{half dozen}

• Ability of a product property:

When a product of bases is raised to some power, the bases will possess the power separately.

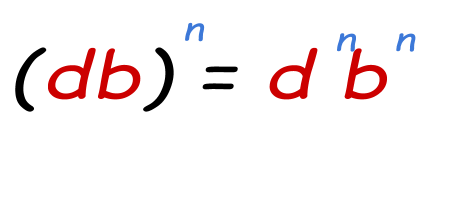
Example: Simplify (4*5)²

4 ^two * v ² =16* 25

= 400

• Ability of a Caliber Property:

Information technology is the same equally the power of a product holding. Power belongs separately to both the numerator and denominator.

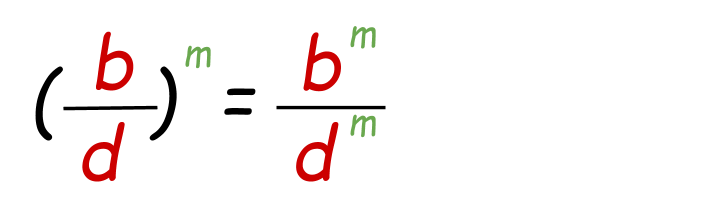
Example: Solve (two/3)^two

(ii/3)²=ii² / 3ⁱⁱ

2²/ 3²=4/nine

sunlablight.blogspot.com

Source: https://www.meracalculator.com/math/exponents.php

Share this post